A ball of mass 1 kg projected with velocity 20 m/s from the origin of an XY coordinate axes at an angle 30° with horizontal. The change in momentum in 1 s is:
1.
2.
3.
4.
Assertion: A safe turn by a cyclist should neither be too fast nor too sharp.
Reason: This is so because it increases the chances of skidding.
1. both Assertion and Reason are true and the reason is the correct explanation of the assertion.
2. both Assertion and Reason are true but the reason is not the correct explanation of the assertion.
3. Assertion is a true statement but Reason is false.
4. both Assertion and Reason are false statements.
A motorcyclist is maintaining constant speed while going on an overbridge of radius R. When the motorcycle is ascending on the overbridge, the normal reaction on it
1. Increases
2. Remains the same
3. Decreases
4. Initially increases upto 45° with vertical and then decreases
A bucket full of water tied with the help of a \(2\) m long string performs a vertical circular motion. The minimum angular velocity of the bucket at the uppermost point so that water will not fall will be:
1. \(2\sqrt{5}\) rad/s
2. \(\sqrt{5}\) rad/s
3. \(5\) rad/s
4. \(10\) rad/s
A mass of \(4\) kg is suspended as shown in the figure with the help of massless inextensible string \(A\).
Another identical string, \(B\), is connected at the lower end of the block. When a sudden pulling downward jerk slightly greater than the breaking strength of \(A\) and \(B\) is given to string \(B\), then:
| 1. | String \(A\) will break. |
| 2. | String \(B\) will break. |
| 3. | Both \(A\) and \(B\) will break simultaneously. |
| 4. | Both \(A\) and \(B\) will never break. |
A \(0.5\) kg body experiences a force \(F=(2+3x^2)\) N, where \(x\) in metres is the displacement from the origin. If it is released to move along the \(X\)-axis from the origin, then its initial acceleration is:
| 1. | \(2~\text{m/s}^2\) | 2. | \(10~\text{m/s}^2\) |
| 3. | \(4~\text{m/s}^2\) | 4. | zero |
On the application of an impulsive force, a sphere of mass \(500\) grams starts moving with an acceleration of \(10\) m/s2. The force acts on it for \(0.5\) s. The gain in the momentum of the sphere will be:
1. \(2.5\) kg-m/s
2. \(5\) kg-m/s
3. \(0.05\) kg-m/s
4. \(25\) kg-m/s
What will be the reading of the spring balance in the given setup? (take \(g=10~\text{m/s}^2\) )

1. \(60~\text N\)
2. \(40~\text N\)
3. \(50~\text N\)
4. \(80~\text N\)
What is the velocity of the block when the angle between the string and the horizontal is \(30^\circ\) as shown in the diagram?

1. \(v_B=v_P\)
2. \(v_B=\frac{v_P}{\sqrt{3}}\)
3. \(v_B=2v_P\)
4. \(v_B=\frac{2v_P}{\sqrt{3}}\)
In the figure shown the horizontal surface is smooth and the strings are inextensible and the massless pulleys are light. If the acceleration of block \(1\) kg is a, then acceleration of \(3\) kg is:
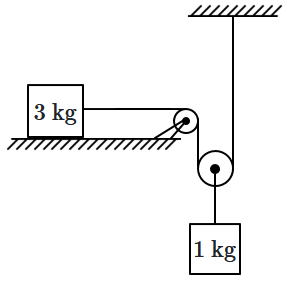
1. \(0\)
2. \(2a\)
3. \(\dfrac{a}{2}\)
4. \(\dfrac{a}{4}\)

