A wheel with a radius of 20 cm has forces applied to it as shown in the figure. The torque produced by the forces of 4 N at A, 8N at B, 6 N at C, and 9N at D, at the angles indicated, is:

1. 5.4 N-m anticlockwise
2. 1.80 N-m clockwise
3. 2.0 N-m clockwise
4. 3.6 N-m clockwise

1. 5.4 N-m anticlockwise
The moment of inertia of a thin rectangular plate ABCD of uniform thickness about an axis passing through the centre O and perpendicular to the plane of the plate is
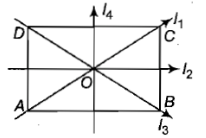
1.
2.
3.
4.
The centre of a wheel rolling on a plane surface moves with a speed \(v_0.\) A particle on the rim of the wheel at the same level as the centre will be moving at speed:
1. zero
2. \(v_0\)
3. \(\sqrt{2}v_0\)
4. \(2v_0\)
Moment of inertia of a uniform cylinder of mass M, radius R and length l about an axis passing through its center and normal to its axis would be
1.
2.
3.
4.
A particle moves along a circle of radius with constant tangential acceleration. If the velocity of the particle is 80 m/s at the end of the second revolution after motion has begin, the tangential acceleration is
1. 640
2. 160
3. 40
4. 40
If the radius of the earth is suddenly contracted to half of its present value, then the duration of the day will be of:
| 1. | 6 hours | 2. | 12 hours |
| 3. | 18 hours | 4. | 24 hours |
A wheel has angular acceleration of 3.0 rad/ and an initial angular speed of 2.00 rad/sec. In a time of 2 sec it has rotated through an angle (in radian) of
1. 10
2. 12
3. 4
4. 6
If a force acts on a body at a point away from the centre of mass, then
1. Linear acceleration changes
2. Angular acceleration changes
3. Both change
4. None of these
If the linear density of a rod of length \(3 \text m\) varies as \(\lambda= \text{2+x} \), then the position of the center of mass of the rod is at a distance of:
1. \({7 \over 3}m\)
2. \({10 \over 7}m\)
3. \({12\over 7}m\)
4. \({9 \over 7}m\)
A sphere can not roll on:
1. a smooth horizontal surface
2. a rough horizontal surface
3. a smooth inclined surface
4. a rough inclined surface
