Ncert Exercises & Solutions

4.15 The experimental data for decomposition of N2O5

[2N2O5 → 4NO2 + O2]

in gas phase at 318K are given below:

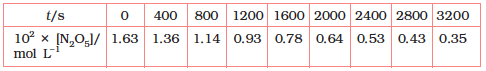
(i) Plot [N2O5] against t.

(ii) Find the half-life period for the reaction.

(iii) Draw a graph between log[N2O5] and t.

(iv) What is the rate law ?

(v) Calculate the rate constant.

(vi) Calculate the half-life period from k and compare it with (ii).

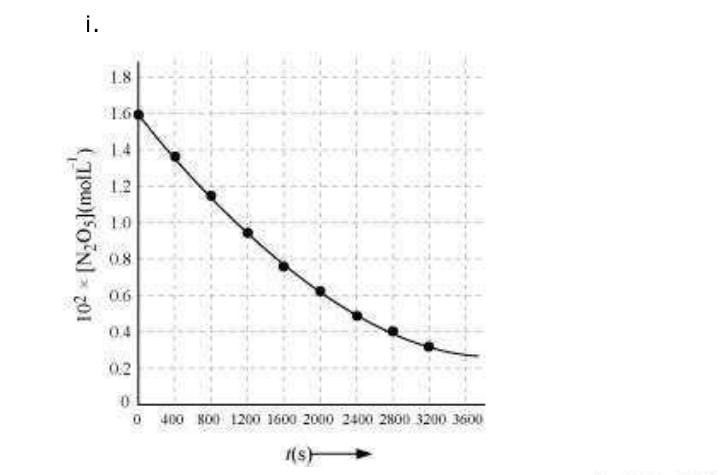
(ii) Time corresponding to the concentration, $\frac{1.630 \times 10^{2}}{2} m o l L^{- 1} = 81.5 m o l L^{- 1},$ is the half
life. From the graph, the half life is obtained as 1450 s.

t(s)	$10^{2} \times {[N_{2} O_{5}]}^{/} m o l L^{- 1}$	log $[N_{2} O_{5}]$
0	1.63	$-$ 1.79
400	1.36	$-$ 1.87
800	1.14	$-$ 1.94
1200	0.93	$-$ 2.03
1600	0.78	$-$ 2.11
2000	0.64	$-$ 2.19

2400	0.53	$-$ 2.28
2800	0.43	$-$ 2.37
3200	0.35	$-$ 2.46

(iv) The given reaction is of the first order as the plot, $\log [N_{2} O_{5}] v / s t,$ is a straight line.
Therefore, the rate law of the reaction is

Rate= K $[N_{2} O_{5}]$

(v) From the plot,

$\log [N_{2} O_{5}]$
$S lo p e = \frac{- 2.46 - (- 1.79)}{3200 - 0}$
$= \frac{- 0.67}{3200}$
$A g a i n, s l o p e o f t h e p l o t loglog [N_{2} O_{5}] / s t i s g i v e n b y$
$- \frac{K}{2.303}$
$T h e r e f o r e, w e o b t a i n,$
$- \frac{K}{2.303} = \frac{0.67}{3200}$
$\Rightarrow K = 4.82 \times 10^{- 4} s^{- 1}$

(vi) Half-life is given by,

$t_{1 / 2} = \frac{0.693}{K}$
$= \frac{0.693}{4.82 \times 10^{- 4}} s$
$= 1.438 \times 10^{3} s$
$= 1438 s$

This value, 1438 s, is very close to the value that was obtained from the graph.

NEET Information

courses

Company

Botany Questions

Chemistry Questions

Physics Questions

Zoology Questions