Ncert Exercises & Solutions

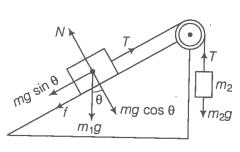
Mass $m_{1}$ moves on a slope making an angle $θ$ with the horizontal and is
attached to mass $m_{2}$ by a string passing over a frictionless pulley as
shown in the figure. The coefficient of friction between $m_{1}$ and the sloping
surface is $μ$ .

$\begin{array}{l} (a) If m_{2} > m_{1} \sin θ, the body will move up the plane. \end{array}$
(b) If m₂>m₁(sin⁡θ+μcos⁡θ), the body will move up the plane.
(c) If m₂<m₁(sin⁡θ+μcos⁡θ), the body will move up the plane.
(d) If m₂<m₁(sin⁡θ−μcos⁡θ), the body will move down the plane.

Which of the following statement/s is/are true?

1. (a, d)

2. (a, c)

3. (c, d)

4. (b, d)

(b, d) Hint: Apply Newton's laws of motion.

Let

m_{1}

moves up the plane, Different forces involved are shown in the diagram.

\begin{array}{l} N = Normal reaction \\ f = Frictional force \\ T = Tension in the string \\ f = μN = {μm}_{1} gcos θ \end{array}

Step 1: Find if the second body moves down.

\begin{array}{l} For the system (m_{1} + m_{2}) to move up \\ \begin{matrix} m_{2} g - (m_{1} gsin θ + f) > 0 \\ m_{2} g - (m_{1} gsin θ + {μm}_{1} gcos θ) > 0 \\ m_{2} > m_{1} (\sin θ + μcos θ) \end{matrix} \end{array}

Hence, option (b) is correct.

Step 2: Find if the first body moves down.
Let the body moves down the plane, in this case, f acts up the plane.

Hence,

\begin{array}{l} m_{1} gsin θ - f > m_{2} g \\ \Rightarrow m_{1} gsin θ - {μm}_{1} gcos θ > m_{2} g \\ \Rightarrow m_{1} (\sin θ - μcos θ) > m_{2} \\ \Rightarrow m_{2} < m_{1} (\sin θ - μcos θ) \end{array}

Hence, option (d) is correct.

NEET Information

courses

Company

Botany Questions

Chemistry Questions

Physics Questions

Zoology Questions