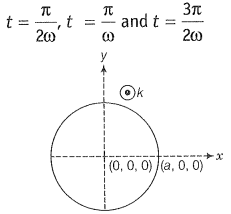
A magnetic field in a certain region is given by and a coil of radius a with resistance R is placed in the x-y plane with its centre at the origin in the magnetic field (figure). Find the magnitude and the direction of the current at (a, 0, 0) at

© 2025 GoodEd Technologies Pvt. Ltd.