25 October - Ray Optics Contact Number: 9667591930 / 852752171825 October - Ray Optics Contact Number: 9667591930 / 8527521718One face of a rectangular glass plate 6 cm thick is silvered. An object held 8 cm in front of the first face, forms an image 10 cm behind the silvered face. The refractive index of the glass is [Consider that light ray returns back in the first medium]
(1) 0.4
(2) 0.8
(3) 1.5
(4) 1.6
In the situation shown, the incident monochromatic ray retraces its path after its incidence on the silvered surface. The speed of light inside the prism will be
1.
2.
3.
4.
Two point light sources are 24 cm apart. Where should a convex lens of focal length 9 cm be put in between them from one source so that the images of both the sources are formed at the same place?
| 1. | 6 cm | 2. | 9 cm |
| 3. | 12 cm | 4. | 15 cm |
The focal length of the objective lens and the eye lens is 4 mm and 25 mm respectively in a compound microscope. The distance between objective and eyepiece lens is 16 cm. Find its magnifying power for relaxed eye position-
1. 32.75
2. 327.5
3. 0.3275
4. None of the above
The distance between a convex lens and a plane mirror is 10 cm. The parallel rays incident on the convex lens, after reflection from the mirror form image at the optical centre of the lens. Focal length of the lens will be:
| 1. | 10 cm | 2. | 20 cm |
| 3. | 30 cm | 4. | Cannot be determined |
The graph between u and v for a convex mirror is:
| 1. |  |
2. |  |
| 3. |  |
4. |  |
An astronomical telescope has angular magnification of 40 in its normal adjustment. If focal length of eyepiece is 5 cm, the length of the telescope is:
1. 190 cm
2. 200 cm
3. 205 cm
4. 210 cm
A lens of focal length \(f_{a}\) in air consists of a glass of refractive index \(\mu_{g}.\) If \(f_{l}\) is its focal length in a liquid of refractive index \(\mu_{l},\) then for \(\mu_{l}=\mu_{g}\)
1. \(f_{l}=0\)
2. \(\infty >f_{l}>f_{a}\)
3. \(0<f_{l}<f_{a}\)
4. \(f_{l}=\infty \)
A square of side 3 cm is placed at a distance of 25 cm from a concave mirror of focal length 10 cm. The centre of the square is at the axis of the mirror and the plane is normal to the axis. The area enclosed by the image of the square is:
| 1. | \(4 \mathrm{~cm}^2 \) | 2. | \(6 \mathrm{~cm}^2 \) |
| 3. | \(16 \mathrm{~cm}^2 \) | 4. | \(36 \mathrm{~cm}^2 \) |
An object is placed at a distance of 20 cm from the thin lens as shown in the figure. The points and denote centres of curvature. The magnification produced by the lens is (Refractive index of glass is 1.5)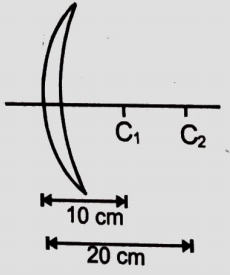
1. -2
2. +2
3. -1/2
4. +1/2
In a glass () sphere of radius 10 cm, there is an air bubble B at distance 5 cm from C. The distance of bubble from the surface of the sphere (i.e. point A) as observed from point P in the air will be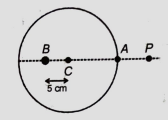
(1) 4.5 cm
(2) 20.0 cm
(3) 9.37 cm
(4) 6.67 cm
A man runs towards a stationary plane mirror with a velocity 15 m/s. The magnitude of the velocity of the man relative to his image is
1. 15 m/s
2. 30 m/s
3. 45 m/s
4. Zero
If \(C_1,~C_2 ~\mathrm{and}~C_3\) are the critical angle of glass-air interface for red, violet and yellow color, then:
| 1. | \(C_3>C_2>C_1\) | 2. | \(C_1>C_2>C_3\) |
| 3. | \(C_1=C_2=C_3\) | 4. | \(C_1>C_3>C_2\) |
A convex lens made of 5 different transparent materials is used to make an image of an object placed in front of it. The number of images formed will be :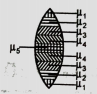
(1) 2
(2) 3
(3) 4
(4) 5
A point source of light B is placed at a distance L in front of the centre of a mirror of width d hung vertically on a wall. A man walks in front of the mirror along a line parallel to the mirror at a distance 2L from it as shown. The greatest distance over which he can see the image of the light source in the mirror is
(1) d/2
(2) d
(3) 2d
(4) 3d
